Potential energy of interaction of point charges
Let two point charges q 1 and q 2 be in vacuum at a distance r from each other. It can be shown that the potential energy of their interaction is given by the formula:
W \u003d kq 1 q 2 / r (3)
We accept formula (3) without proof. Two features of this formula should be discussed.
First, where is the zero level of potential energy? After all, potential energy, as can be seen from formula (3), cannot vanish. But in fact, the zero level exists, and it is at infinity. In other words, when the charges are located infinitely far from each other, the potential energy of their interaction is assumed to be zero (which is logical - in this case, the charges already "do not interact"). Secondly, q 1 and q 2 are again the algebraic values \u200b\u200bof the charges, i.e. charges taking into account their sign.
For example, the potential energy of interaction of two charges of the same name will be positive. Why? If we let them go, they will start to accelerate and move away from each other.
Their kinetic energy increases, and therefore the potential energy decreases. But at infinity, potential energy vanishes, and since it decreases to zero, it means that it is positive.
But the potential energy of interaction of opposite charges is negative. Indeed, let's remove them a very large distance from each other - so the potential energy is zero - and let go. The charges will begin to accelerate, drawing closer, and the potential energy decreases again. But if she was zero, then where did she go? Only towards negative values.
Formula (3) also helps to calculate the potential energy of a system of charges if the number of charges is more than two. To do this, sum the energies of each pair of charges. We will not write out a general formula; we better illustrate what was said with a simple example, depicted in Fig. 8
Fig. 8. The interaction of the three charges
If the charges q 1, q 2, q 3 are at the vertices of the triangle with sides a, b, c, then the potential energy of their interaction is:
W \u003d kq 1 q 2 / a + kq 2 q 3 / b + kq 1 q 3 / c
Potential
From the formula W \u003d - qEx, we see that the potential charge energy q in a uniform field is directly proportional to this charge. We see the same thing from the formula W \u003d kq 1 q 2 / r potential energy of the charge q 1 located in the field of point charge q 2 is directly proportional to the value of charge q 1. It turns out that this is a general fact: the potential energy W of the charge q in any electrostatic field is directly proportional to the quantity q:
The value of q no longer depends on the charge, is a characteristic of the field and is called the potential:
So, the potential of a uniform field E at a point with an abscissa x is:
Recall that the X axis coincides with the field strength line. We see that with increasing x, the potential decreases. In other words, the field strength vector indicates the direction of decreasing potential. For the potential of the field of a point charge q at a distance r from it, we have:
The unit of potential is the well-known volt. From formula (5) we see that B \u003d J / C.
So, now we have two characteristics of the field: power (tension) and energy (potential). Each of them has its advantages and disadvantages. Which characteristic is more convenient to use depends on the specific task.
If an electric body acts on electrically charged bodies, then it is able to do the work of moving charged bodies. The electrostatic field created by a point charge is central, that is, the force acting on a point charge in such a field is directed along a straight line connecting the charge source and the test charge. Earlier, we showed that any central force is potential, that is, the work of this force does not depend on the shape of the trajectory, but is determined only by the initial and final position of the body.
We briefly recall the proof of this most important statement. Let the point test charge q move in the central field created by the motionless charge Q (Fig. 174). The force acting on the test charge is determined by the law of Coulomb
Where is the vector drawn from the charge of the source Q to point A, at which the test charge is located. When a charge moves along arcs of circles centered on a charge Q (for example, along arcs AB, CD), the work of electric force is zero, so the force and displacement vectors are mutually perpendicular. When moving in the radial direction (for example, along the segments BC, DE), the operation depends only on the initial and final distances to the source charge. So the work of the electrostatic field when moving along the segments DE and D1E1 are obviously equal. The most beautiful proof of this statement is related to the symmetry of the field - we turn our system around the axis passing through the source so that the segment D1E1 coincides with the segment DE - the distribution of the field does not change, why should the field work?
Since the principle of superposition is valid for electrostatic field strength, any electrostatic field is potential. Indeed, let a point charge q be in an electric field created by a system of fixed point charges Q1, Q2, ..., QN. When the charge moves to a small displacement vector, by definition, the electric field will do the job where
The resulting force acting on the moving charge q is equal to the sum of the forces acting on the side of each of the stationary point charges Qk. The work of this force can be calculated by the formula
In order to calculate the work along the final section of the trajectory, it is necessary to divide the path into small sections (Fig. 175), then using formula (1) to calculate the work in each small section, and then sum them up
. (2) In fact, this amount is double, since each resulting force is the sum of the forces in accordance with formula (1). Note that in formula (2), the resulting force changes, as it is calculated at different points on the trajectory.
As we showed earlier, the work of the electric field of a point charge does not depend on the shape of the trajectory, that is, each term from formula (1) does not depend on the shape of the trajectory, therefore, the entire sum does not depend on the shape of the trajectory. Thus, any electrostatic field is potential.
Therefore, for a point charge located in an electrostatic field, one can introduce the potential interaction energy U (x, y, z). This function has the following physical meaning: the electric field when moving a point charge from one point with coordinates (x1, y1, z1) to another, with coordinates (x2, y2, z2) is equal to the change in potential energy taken with the opposite sign:
. (3) The change of sign in this definition is quite logical: if the electric field has done a positive job (A\u003e 0), then its energy decreases (ΔU< 0). Для вычисления работы силы взаимодействия между двумя точечными заряженными телами достаточно подсчитать эту работу при движении вдоль радиального отрезка при изменении расстояния от r1 до r2 (Рис. 176). Если построить зависимость силы взаимодействия между зарядами от расстояния r между телами, тогда площадь под графиком этой зависимости в указанных пределах и будет равна искомой работе (Рис. 177). Зависимость силы электростатического взаимодействия от расстояния аналогична силе гравитационного взаимодействия, с одним существенным отличием: гравитационная сила всегда есть сила притяжения, а электрическая может быть как силой притяжения, так и силой отталкивания. В частности два положительных заряда отталкиваются. Поэтому выражение для работы электрического поля, будет аналогично формуле для работы гравитационной силы, но иметь противоположный знак
This work is equal to a decrease in the potential energy of interaction, that is, from this expression we can determine the expression for the potential energy of the electrostatic interaction of two point charges. (4) With this definition, the potential energy of interaction of two charges of the same sign is positive and tends to zero at an infinite distance between the bodies. The force of interaction of charges of opposite signs is directed in the opposite direction, so the work of this force with increasing distance between charges will be negative. However, we do not need to make any additional reservations, since formula (4) automatically takes into account the signs of charges - if the charges are opposite, then their product (respectively, energy) is negative. The sign of the potential energy of interaction of charges has a very clear meaning. The charges of the same sign repel, therefore, when they “scatter” over an infinitely large distance, the electric field will do a positive job - therefore, initially the system of these charges has the ability to do the work, therefore its energy is positive, when the charges move away from each other, their energy decreases to zero. Charges of opposite signs are attracted, in order to remove them to an infinitely large distance, external forces must do positive work. In this case, the energy of a pair of charges should increase, therefore, initially it is negative, and when the charges are removed from each other, it increases to zero. In general, the usual situation - attraction corresponds to negative energy, and repulsion - positive. We only note that such evidence is valid only when choosing a zero level of potential energy at infinity. Formula (4) determines the potential energy of interaction of two point charged bodies. The values \u200b\u200bof the charges of the bodies Q and q enter, as one would expect, in this formula symmetrically. The division of charges into a source charge and a test charge is conditional, they can be completely interchanged. Therefore, this formula is preferable to write in a symmetric form: the interaction energy of two point charges q1 and q2 is equal to, (5) and it makes sense to work done by the field with increasing distance between charges from r to infinity, regardless of whether the first charge moves, or the second , or both charges move, finally, regardless of the trajectories of motion of both charges. Further, it cannot be said which particular charge this energy “belongs” to, we will show later that the interaction energy of the charges is part of the energy of the electrostatic field itself, that is, it is “smeared” throughout the space where the field created by these charges exists. If the system consists of more than two charges, then to calculate the interaction energy of these charges, it is necessary to sum the interaction energies of all pairs of charges
here Uik is the interaction energy of the charges qi and qk located at a distance rik from each other (Fig. 178).
40 Question:
Electrostatic field - el. field of motionless charge.
The fel acting on the charge moves it, making a rabo. In a uniform electric field, Fel \u003d qE is a constant
The work of the field (electric power) does not depend on the shape of the trajectory and on a closed path \u003d zero.
POTENTIAL ENERGY OF A CHARGED BODY IN A HOMOGENEOUS ELECTROSTATIC FIELD
Electrostatic energy is the potential energy of a system of charged bodies (because they interact and are able to do the job).
Since the work of the field does not depend on the shape of the trajectory, then simultaneously
comparing the work formulas, we obtain the potential charge energy in a uniform electrostatic field
If the field does a positive job (along the lines of force), then the potential energy
charged body decreases (but according to the law of conservation of energy, kinetic energy increases) and vice versa.
ELECTROSTATIC FIELD POTENTIAL
Energy characteristic el. fields.
Equal to the ratio of the potential energy of a charge in the field to this charge.
The scalar quantity that determines the potential energy of the charge at any point el. fields.
The potential value is considered relative to the selected zero level.
DP (or otherwise VOLTAGE)
This is the potential difference at the initial and final points of the charge path.
The voltage between two points (U) is equal to the potential difference of these points and is equal to the work of the field in moving a unit charge.
COMMUNICATION BETWEEN FIELD TENSION AND DIFFERENCE OF POTENTIALS
The work of the forces of the electric field created by the charge to move the charge  from point 1 to point 2 is equal to:
from point 1 to point 2 is equal to:
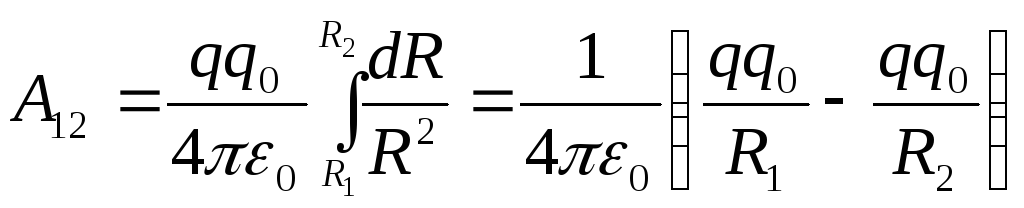 .
.
The work of the forces of the conservative field is equal to the loss of potential energy:
 ,
,
then the potential charge energy  in charge field
in charge field  is equal to:
is equal to:
 .
.
The value of the constant is chosen so that when the charge is removed to infinity (i.e., when 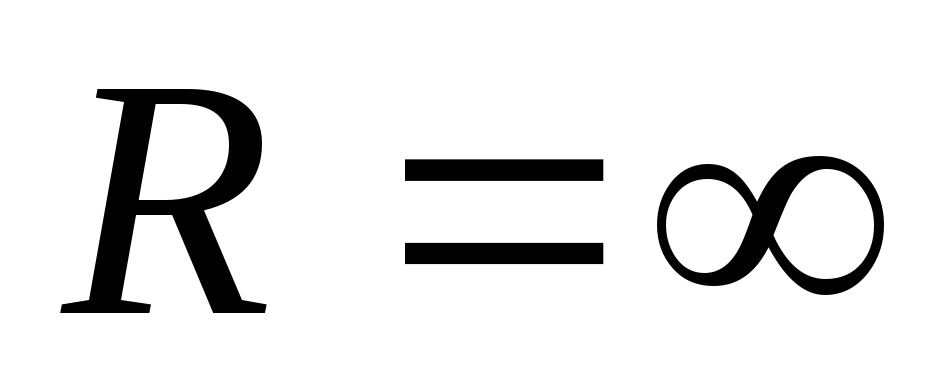 ) potential energy would vanish, therefore
) potential energy would vanish, therefore
 .
.
It is clear that different test charges 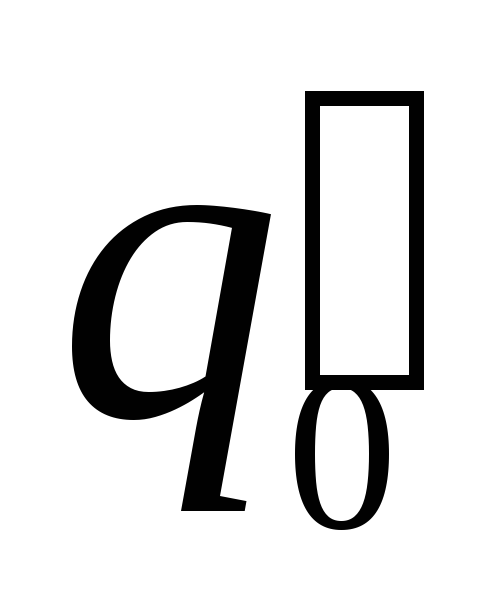 and
and 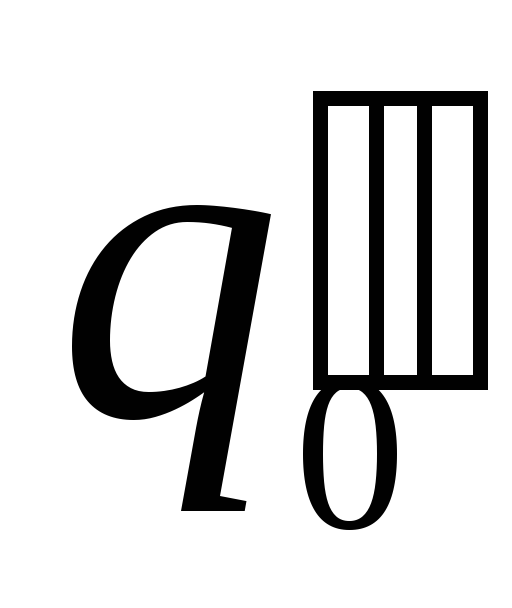 at the same point the fields will have different potential energy
at the same point the fields will have different potential energy  and
and 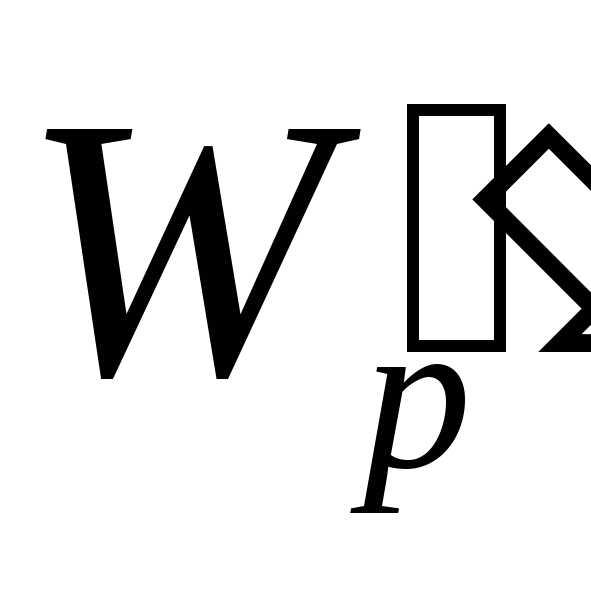 . However attitude
. However attitude 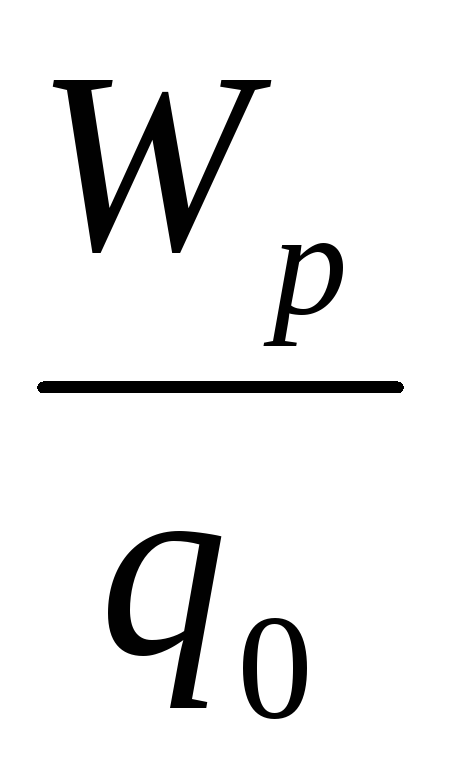 for all test charges will be the same. Value
for all test charges will be the same. Value

called the potential of the electric field and is its energy characteristic. The potential of the field of a point charge is
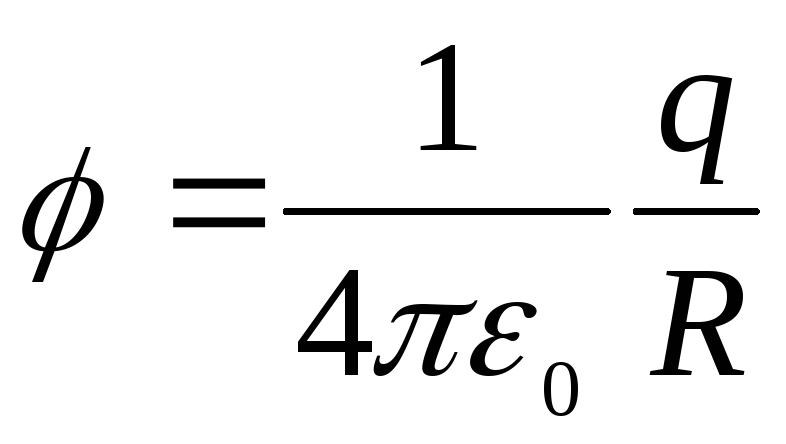 .
.
If the field is created by the system  point charges then
point charges then
 ,
,
where  - distance from charge
- distance from charge  to the initial charge position
to the initial charge position  ,
, - distance from charge
- distance from charge  to the final charge position
to the final charge position  (charge
(charge  moves by the forces of the field).
moves by the forces of the field).
Then the potential energy of the charge  in the field of the charge system:
in the field of the charge system:
 ,
,
and potential 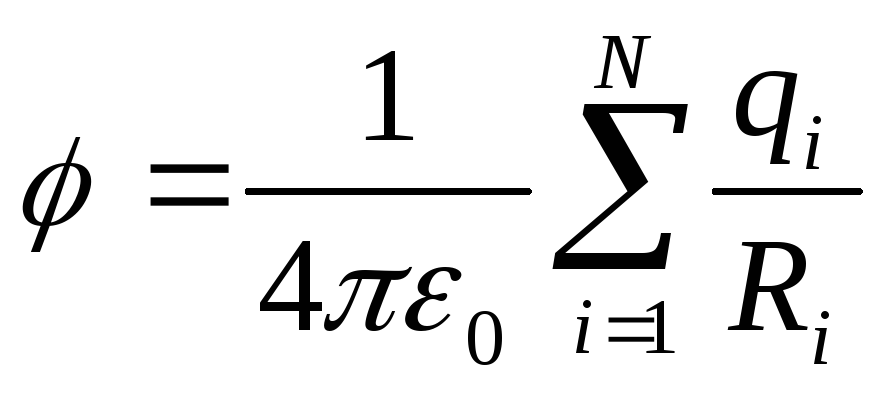
the potential of the field created by the system of charges is equal to the algebraic sum of the potentials created by each of the charges separately.
Knowing the potential, you can find the potential energy of the charge  in an electric field:
in an electric field:
 .
.
Field work on charge:
The work is equal to the loss of potential times the charge.
If the charge is removed from the point to infinity, then the work of the field forces is equal to
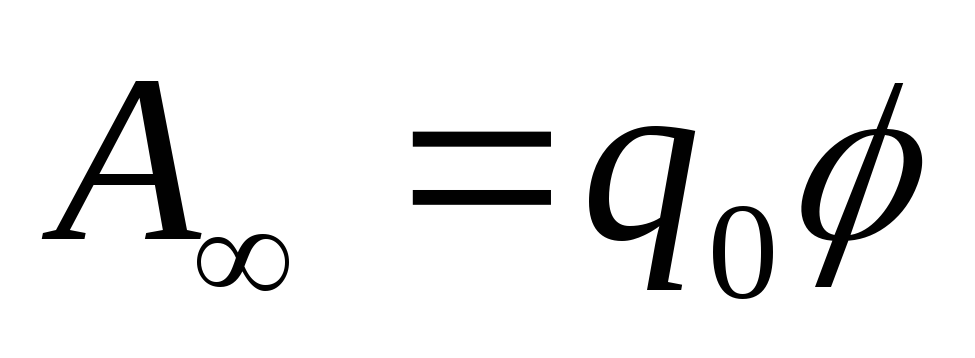
therefore, the potential is numerically equal to the ratio of the work that the field forces perform on a positive charge when it is removed from a given point to infinity, to the magnitude of this charge. Potential is measured in volts: 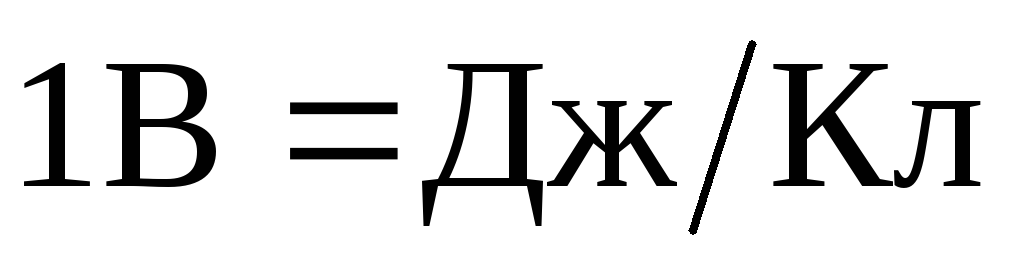 .
.
1.5. The relationship between tension and potential
The electric field can be described either using the vector quantity  (power characteristic), or using a scalar
(power characteristic), or using a scalar  (energy characteristic). Strength is associated, as you know, with potential energy:
(energy characteristic). Strength is associated, as you know, with potential energy:
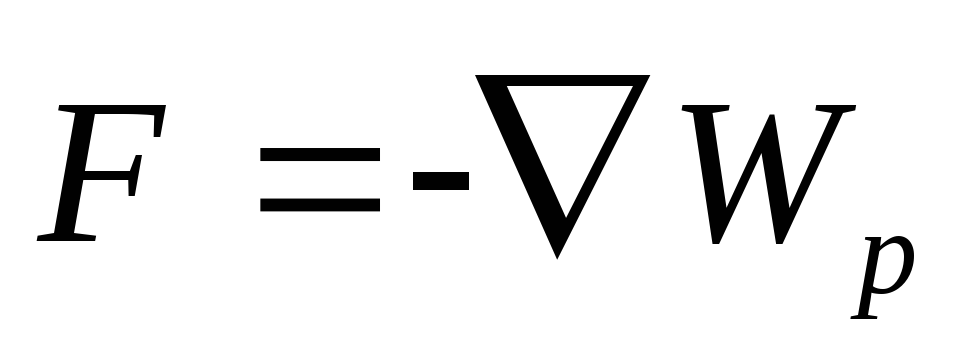 ,
,
where  - Nabla operator,
- Nabla operator,  .
.
For a charged particle in an electric field: 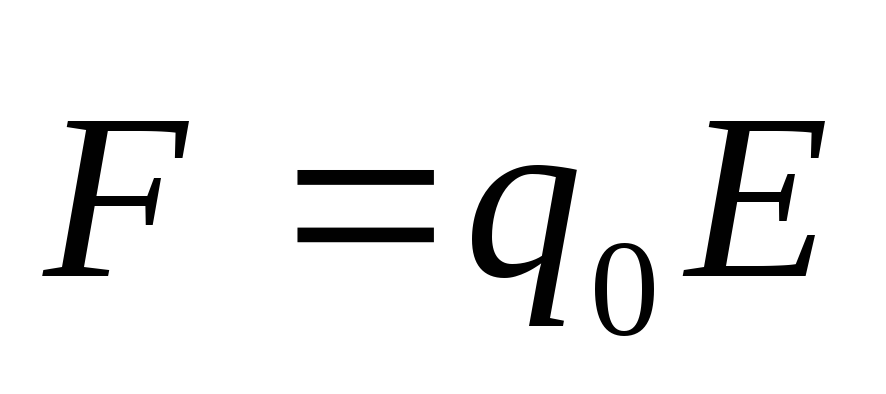 ,
, then
then  ,
, then
then 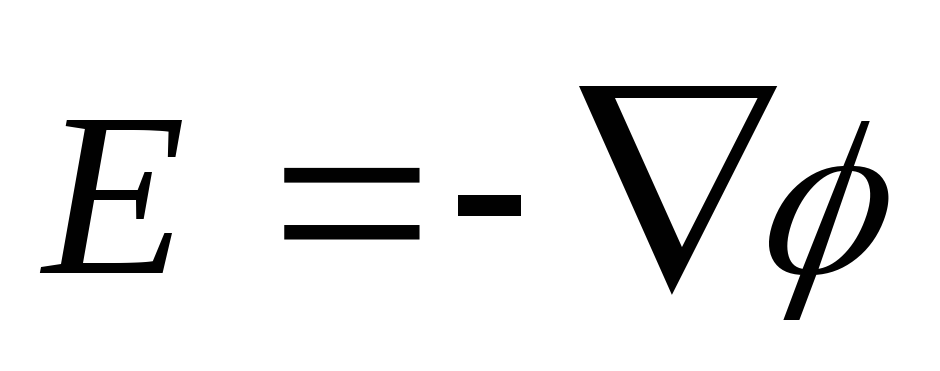 - the relationship of tension and potential, or
- the relationship of tension and potential, or 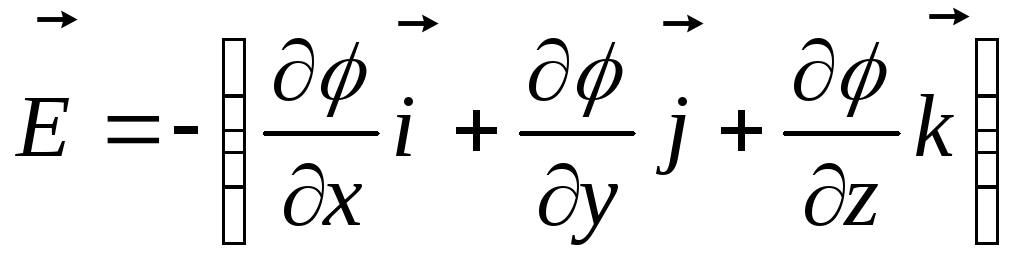 , or
, or 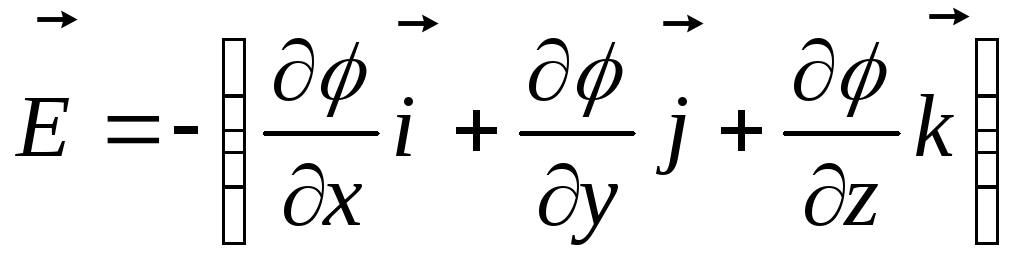 , or
, or  -
vector projection
-
vector projection  in any direction
in any direction  equal to the rate of decrease of potential
equal to the rate of decrease of potential  along the direction
along the direction
 ,
or
,
or
 .
.
Since the gradient of the potential is directed toward its increase, and the numerical value of the gradient is a measure of the speed of this increase, it can be said that the electric field strength is a measure of the rate of decrease of the potential, or, simply, that it is equal to the decrease of the potential.
Back to the definition of the field: 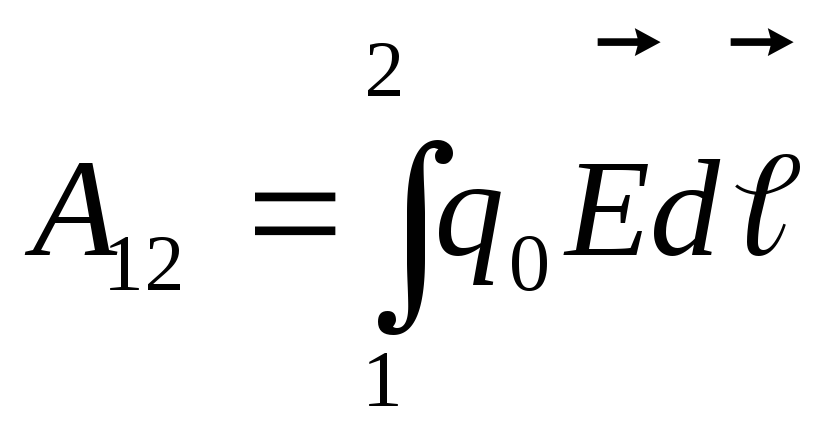 ,
,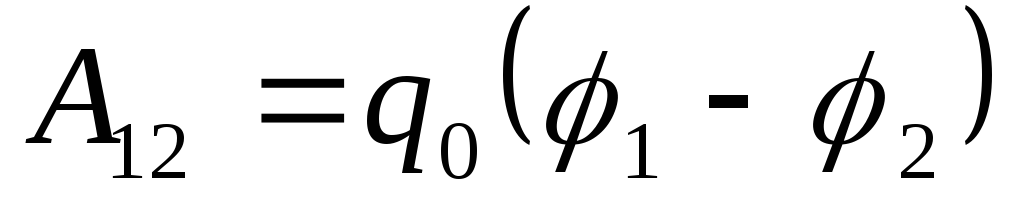 , hence the circulation of the vector
, hence the circulation of the vector
 on the plot 1 \u003d 2 is
on the plot 1 \u003d 2 is  . The integral can be taken along any line connecting points 1 and 2, since the work does not depend on the path.
. The integral can be taken along any line connecting points 1 and 2, since the work does not depend on the path.
To bypass in a closed loop: 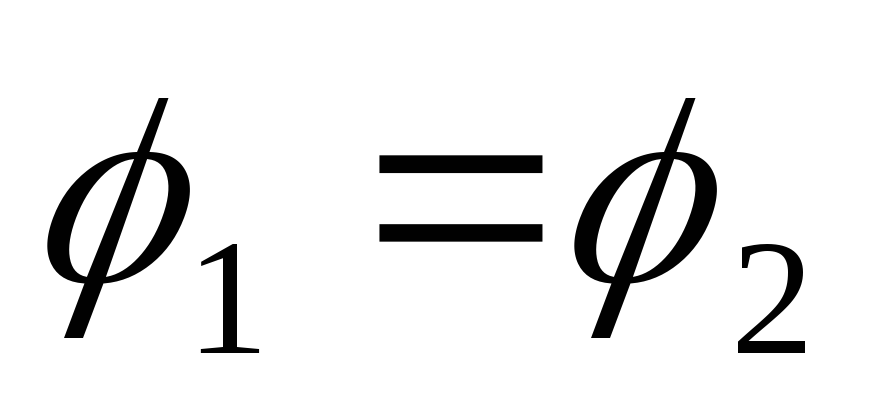 and
and 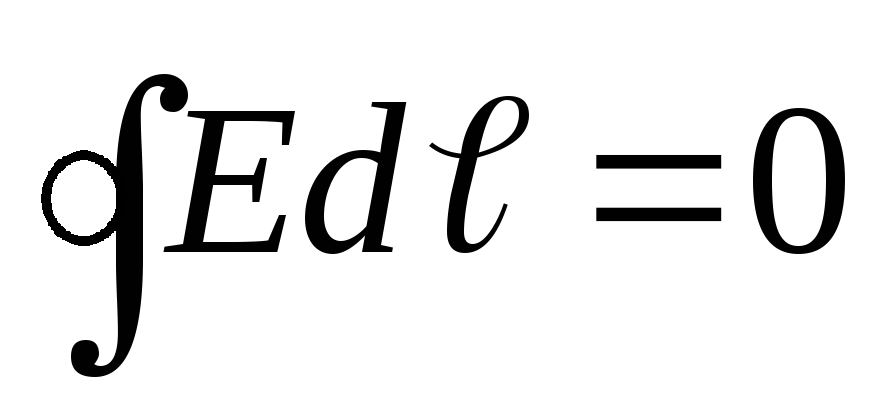 - came to a theorem on the circulation of the electrostatic field intensity vector.
- came to a theorem on the circulation of the electrostatic field intensity vector.
1.6. Equipotential surfaces
An imaginary surface, all points of which have the same potential, is called an equipotential surface:
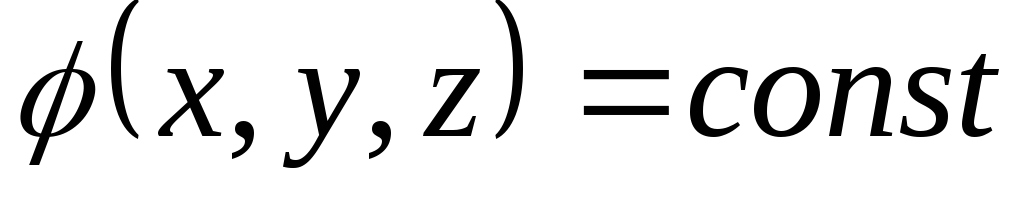
Equipotential surface equation.
When moving along an equipotential surface onto a segment  potential does not change
potential does not change  . Thus, the component of the vector tangent to the surface
. Thus, the component of the vector tangent to the surface  equal to zero. Then the vector
equal to zero. Then the vector  directed normal to the equipotential surface at each of its points, and the lines of tension at each point are perpendicular to equipotential surfaces.
directed normal to the equipotential surface at each of its points, and the lines of tension at each point are perpendicular to equipotential surfaces.
 If equipotential surfaces are constructed in such a way that the potential difference for two adjacent surfaces is the same, then the density of the equipotential surfaces can be used to judge the field strength. Indeed, the thicker the equipotential surfaces, the greater
If equipotential surfaces are constructed in such a way that the potential difference for two adjacent surfaces is the same, then the density of the equipotential surfaces can be used to judge the field strength. Indeed, the thicker the equipotential surfaces, the greater  , the more
, the more  .
.
For a homogeneous field, equipotential surfaces are a system of equally spaced planes perpendicular to the direction of the field.
Consider the equipotential surface of a point charge. Potential of a point charge (Fig. 1.4)
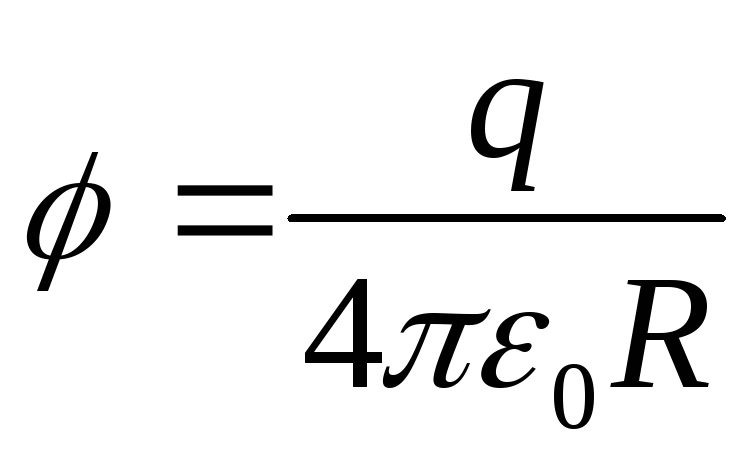 .
.
Thus, the equipotential surface of this charge will be a sphere of radius 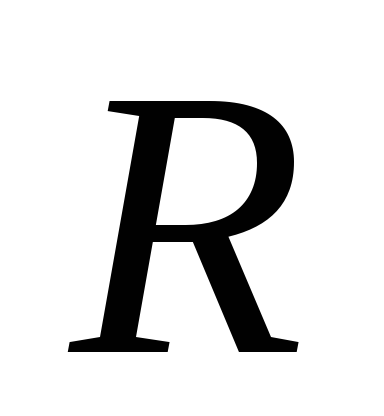 centered at the point of charge. The lines of force, as we established earlier, diverge radially from the charge if it
centered at the point of charge. The lines of force, as we established earlier, diverge radially from the charge if it  , or converge to charge if it is “-”. That is a vector
, or converge to charge if it is “-”. That is a vector  perpendicular to equipotential surfaces.
perpendicular to equipotential surfaces.
Potential charge energy in an electric field.The work done by the forces of an electric field when moving a positive point charge qfrom position 1 to position 2, imagine as a change in the potential energy of this charge:
where W n1 and W n2 - potential charge energies q in positions 1 and 2. With a small charge movement q in the field created by a positive point charge Q, the change in potential energy is
![]() .
.
With the final movement of the charge q from position 1 to position 2 at distances r 1 and r 2 on charge Q,
If the field is created by a system of point charges Q 1 , Q 2 ,¼ , Q n, then the change in the potential energy of the charge qin this field:
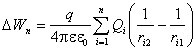 .
.
The above formulas allow you to find only change potential energy of a point charge q, and not the potential energy itself. To determine the potential energy, it is necessary to agree at what point in the field to consider it equal to zero. For potential energy of a point charge qlocated in an electric field created by another point charge Qwe get
![]() ,
,
where C Is an arbitrary constant. Let the potential energy be equal to zero at an infinitely large distance from the charge Q (at r® ¥ ), then the constant C\u003d 0 and the previous expression takes the form
In this case, the potential energy is defined as the work of moving a charge by field forces from a given point to an infinitely remote. In the case of an electric field created by a system of point charges, the potential energy of the charge q:
![]() .
.
Potential energy of a system of point charges.In the case of an electrostatic field, potential energy serves as a measure of the interaction of charges. Let there be a system of point charges in space Q i(i = 1, 2, ... , n) Energy interactions of all n charges is determined by the ratio
![]() ,
,
where r ij -the distance between the respective charges, and the summation is performed so that the interaction between each pair of charges is taken into account once.
Potential of electrostatic field.A field of conservative force can be described not only by a vector function, but an equivalent description of this field can be obtained by determining a suitable scalar value at each of its points. For an electrostatic field, this quantity is electrostatic field potentialdefined as the ratio of the potential energy of the test charge q to the magnitude of this charge, j = W P / q, whence it follows that the potential is numerically equal to the potential energy that a unit positive charge possesses at a given point in the field. The unit for measuring potential is Volt (1 V).
Potential of a point charge field Qin a homogeneous isotropic medium with dielectric constant e :
The principle of superposition.Potential is a scalar function; the principle of superposition is valid for it. So for the field potential of a system of point charges Q 1, Q 2 ¼ , Q n we have
![]() ,
,
where r i - distance from the point of the field with potential jup to charge Q i. If the charge is arbitrarily distributed in space, then
![]() ,
,
where r- distance from elementary volume d x, d y, d z to the point ( x, y, z), where the potential is determined; V - the amount of space in which the charge is distributed.
Potential and work of electric field forces.Based on the determination of the potential, it can be shown that the work of the electric field forces when moving a point charge q from one point of the field to another is equal to the product of the magnitude of this charge by the potential difference at the initial and final points of the path, A \u003dq (j 1
-
j 2
)
.
If, by analogy with potential energy, we assume that at points infinitely remote from electric charges - field sources, the potential is zero, then the work of the electric field forces when the charge moves q from point 1 to infinity can be represented as A ¥
=
qj 1 .
Thus, the potential â at a given point of the electrostatic field is physical quantity numerically equal to the work performed by the forces of an electric field when moving a unit positive point charge from a given point of the field to an infinitely distant: j = A ¥
/ q.
In some cases, the electric field potential is more clearly defined as physical quantity numerically equal to the work of external forces against electric field forces when a unit positive point charge moves from infinity to a given point. The last definition is conveniently written as follows:
In modern science and technology, especially when describing the phenomena occurring in the microworld, a unit of work and energy is often used, called electron volt (eV). This is the work done when moving a charge equal to the charge of an electron between two points with a potential difference of 1 V: 1 eV \u003d 1.60 × 10 - 1 9 Cl × 1 V \u003d 1.60 × 10 - 1 9 J.
Questions
1) Give a definition of the potential of a given point of the field and the potential difference of two points of the field.
2) Give graphs of the dependence of field strength and potential on distance for a uniformly charged spherical surface. Give their explanation and justification.
The energy of a system of point charges as the sum of the energy of pair interaction of charges (and the principle of superposition)
Potential charge energy in an electric field.The work done by the forces of an electric field when moving a positive point charge q from position 1 to position 2, imagine as a change in the potential energy of this charge:
where W n1 and W n2 - potential charge energies q in positions 1 and 2. With a small charge movement q in the field created by a positive point charge Q, the change in potential energy is
![]() .
.
With the final movement of the charge q from position 1 to position 2 at distances r 1 and r 2 on charge Q,
If the field is created by a system of point charges Q 1 , Q 2, ¼, Q n, then the change in the potential energy of the charge q in this field:
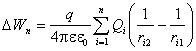 .
.
The above formulas allow you to find only change potential energy of a point charge q, and not the potential energy itself. To determine the potential energy, it is necessary to agree at what point in the field to consider it equal to zero. For potential energy of a point charge qlocated in an electric field created by another point charge Qwe get
![]() ,
,
where C Is an arbitrary constant. Let the potential energy be equal to zero at an infinitely large distance from the charge Q (at r ® ¥), then the constant C \u003d 0 and the previous expression takes the form
In this case, the potential energy is defined as the work of moving the charge by the forces of the field from a given point to an infinitely remote. In the case of an electric field created by a system of point charges, the potential energy of the charge q:
![]() .
.
Potential energy of a system of point charges.In the case of an electrostatic field, potential energy serves as a measure of the interaction of charges. Let there be a system of point charges in space Q i (i = 1, 2, ... ,n) Energy interactions of all n charges is determined by the ratio
![]() ,
,
where r ij - the distance between the respective charges, and the summation is performed so that the interaction between each pair of charges is taken into account once.
Potential of electrostatic field.A field of conservative force can be described not only by a vector function, but an equivalent description of this field can be obtained by determining a suitable scalar value at each of its points. For an electrostatic field, this quantity is electrostatic field potentialdefined as the ratio of the potential energy of the test charge q to the magnitude of this charge, j \u003d W P / q, whence it follows that the potential is numerically equal to the potential energy that a unit positive charge possesses at a given point in the field. The unit for measuring potential is Volt (1 V).
Potential of a point charge field Q in a homogeneous isotropic medium with a dielectric constant e:
The principle of superposition.Potential is a scalar function; the principle of superposition is valid for it. So for the field potential of a system of point charges Q 1, Q 2 ¼, Q n we have
![]() ,
,
where r i is the distance from the point of the field with potential j to the charge Q i . If the charge is arbitrarily distributed in space, then
![]() ,
,
where r - distance from elementary volume d x, d y, d z to the point ( x, y, z), where the potential is determined; V - the amount of space in which the charge is distributed.
Potential and work of electric field forces.Based on the determination of the potential, it can be shown that the work of the electric field forces when moving a point charge q from one point of the field to another is equal to the product of the magnitude of this charge by the potential difference at the initial and final points of the path, A = q (j 1 - j 2). If, by analogy with potential energy, we assume that at points infinitely remote from electric charges - field sources, the potential is zero, then the work of the electric field forces when the charge moves q from point 1 to infinity can be represented as A ¥ = q j 1. Thus, the potential â at a given point of the electrostatic field is physical quantity numerically equal to the work performed by the forces of an electric field when moving a unit positive point charge from a given point of the field to an infinitely distant: j \u003d A ¥ / q. In some cases, the electric field potential is more clearly defined as physical quantity numerically equal to the work of external forces against electric field forces when a unit positive point charge moves from infinity to a given point. The last definition is conveniently written as follows:
In modern science and technology, especially when describing the phenomena occurring in the microworld, a unit of work and energy is often used, called electron volt (eV). This is the work done when moving a charge equal to the charge of an electron between two points with a potential difference of 1 V: 1 eV \u003d 1.60 × 10 -19 C × 1 V \u003d 1.60 × 10 -19 J.
Integral representation of the energy of a continuous charge distribution, c alignment with the case of energy of a system of point charges
Let there be a charge in the volume element. To determine the interaction energy of all elements in volume V, one can use formula (12.4). going in it from the sum to the integral:
Where is the potential created by all charges at the point where the charge is located.
For the first charge, formulas (12.4) and (12.5) seem similar, especially since (12.5) is “derived” from (12.4). However, there is a fundamental difference between them. Formula (12.4) takes into account only the interaction energy between charged balls, but does not take into account the interaction energy between the elements of charges located on each ball. And (12.5) takes into account the first and second.
Given the foregoing, the energy of interaction of charges can be written in the form:
Value is the energy of charged balls, taking into account the interaction of charges with each other on each ball. Own energy depends on the laws of distribution of the charges of the ball and the values \u200b\u200bof the charges. If there is a solitary ball, then.
Then ![]() (12.7)
(12.7)
This means that the self-energy of a point charge is infinity.
But with. This leads to serious difficulties when using the model of point charges.
Electric energy of a charged solitary conductor and capacitor
If a solitary conductor has a charge q, then around it there is an electric field whose potential on the surface of the conductor is equal, and the capacitance is C. We increase the charge by dq. When transferring the charge dq from infinity, work equal to ![]() . But the potential of the electrostatic field of a given conductor at infinity is zero. Then
. But the potential of the electrostatic field of a given conductor at infinity is zero. Then
![]()
When the charge dq is transferred from the conductor to infinity, the electrostatic field forces do the same work. Therefore, as the conductor charge increases by dq, the potential field energy increases, i.e.
![]()
Integrating this expression, we find the potential energy of the electrostatic field of a charged conductor with an increase in its charge from zero to q:
![]()
Applying the relation, we can obtain the following expressions for the potential energy W:
The energy of the electrostatic field, expressed as the volume integral of the vectors of intensity E and electric displacementD .

The expression of the force acting on a conductor immersed in a liquid or gaseous dielectric through the bulk energy density of the electric field near the conductor.
In the presence of a medium, the calculation of the forces acting on conductors and dielectrics is complicated.
First of all, the expression for bulk force becomes unjust, even if we mean the molecular charge density. This is due to the fact that there is an average macroscopic density that does not take into account the polarization of individual molecules. Meanwhile, in a nonuniform electric field, a force acts on a polarized molecule. One could try to average this force over the volume, but such a procedure runs into significant difficulties. We will use the energy method of calculating forces.
Let's consider some typical tasks. We find the force acting on a dielectric ball placed in a weakly inhomogeneous field. The last condition means that the field should change little on the size of the ball. Then the dipole moment of the ball will be approximately the same as in a uniform field: where E is the external field (in the absence of the ball). Since the moment of the ball is proportional to the field, it behaves as a quasielastic dipole and, therefore, its energy in the field. Now we will make a virtual movement of the ball in an external inhomogeneous field and write down the energy balance: ![]() where is the force acting on the ball from the field side:
where is the force acting on the ball from the field side:
![]() (19.1)
(19.1)
i.e., the dielectric is drawn into a strong field. If (weak insulator), then expression (19.1) is valid for an insulator of arbitrary shape, since in this case we can neglect the interaction of individual parts of the insulator, which are polarized independently of each other. Then the volumetric force acting on the dielectric,
![]() (19.2)
(19.2)
i.e., it is determined by a change in the energy density of the electric field when a dielectric is introduced.
In addition to the force acting in the inhomogeneous electric field on the dielectric as a whole, internal stresses also arise in it, called striction forces. Consider a dielectric plate placed in a flat capacitor (Fig. 11.5). It is clear that under the action of striction forces, the plate

Fig. 11.5. To the calculation of striction forces.
stretches along the field. Let's try to calculate the striction forces in this example. We will use the energy method. With a slight stretching of the plate, the change in the field energy consists of two parts. First, in the layer, the field energy in vacuum is replaced by the field energy in the medium. Here is the field in the vacuum gap, which does not change when the dielectric is deformed, since we take the charge on the capacitor unchanged (see above). Secondly, it is necessary to take into account the change in energy in the entire volume of a substance due to a change in its density, on which the dielectric constant depends: ![]() where
where ![]() .The partial derivative is taken here at a constant temperature in order to exclude a temperature dependence. The total energy balance per unit area of \u200b\u200bthe dielectric has the form
.The partial derivative is taken here at a constant temperature in order to exclude a temperature dependence. The total energy balance per unit area of \u200b\u200bthe dielectric has the form
Hence the tension acting on the dielectric,
![]() (19.4)
(19.4)
can be considered as the difference in tension from the outside and from the inside ![]() dielectric, where E is the electric field inside the latter.
dielectric, where E is the electric field inside the latter.
Strictive pressure is usually called the quantity
![]() (19.5)
(19.5)
This pressure does not contribute to the force acting on the dielectric as a whole, provided that it is surrounded by vacuum.
Finally, we consider an arbitrary system of charged bodies immersed in a homogeneous liquid dielectric. As we already know, such an environment weakens the field by a factor of time without changing its configuration. From this, in particular, it follows that the field energy is also several times smaller than in vacuum. This means that the work of moving charges and the forces between bodies also decrease by a factor of one. At first glance, this conclusion seems trivial: once the field decreases by a factor of 1, then the force of its effect on the charge should decrease by the same amount. However, the field in the medium is understood as the average field, whereas the local field acting on the charge depends on the shape of the cavity, i.e., on the shape of the charged body. To understand what is the matter here, let us return to the previous example. Let the dielectric is now liquid and fill the entire capacitor. Nevertheless, we can imagine that between the dielectric and the capacitor plate there is a very
a thin gap in which the field is equal so that all the previous consideration remains valid. In this case, the field pressure directly on the plate is equal to the same as in vacuum, instead of the expected attenuation by a factor of one. This example confirms that the force exerted by the field on the charged body really depends on the shape of the body.
However, a liquid dielectric, as a rule, has mechanical contact with the body and also acts on it with some force, which is given by expression (19.4) in the considered example. Finally, it is necessary to take into account the additional pressure in the liquid, which arises due to the electric field and is equal to the striction pressure (19.5). Thus, the total pressure on the plate
![]() (19.6)
(19.6)
in accordance with energy considerations.
We emphasize once again that such a simple result is obtained only for a liquid homogeneous dielectric. The mechanical contact of conductors with a solid dielectric is, as a rule, uncertain. In addition, internal elastic stresses now depend not on local strictive pressure, but on the forces acting on the entire dielectric.
